Book
-
 The fractional Laplacian uncovered (with D. dos Prazeres and A. Sobral), Brazilian Mathematics Colloquium, vol. 35-10, IMPA, 2025.
The fractional Laplacian uncovered (with D. dos Prazeres and A. Sobral), Brazilian Mathematics Colloquium, vol. 35-10, IMPA, 2025.
Peer-reviewed journal papers
 Improved regularity for a nonlocal dead-core problem (with D. dos Prazeres and J.M. Urbano), submitted.
Improved regularity for a nonlocal dead-core problem (with D. dos Prazeres and J.M. Urbano), submitted.
 Geometric properties of free boundaries in degenerate quenching problems (with D.J. Araújo and J.M. Urbano), submitted.
Geometric properties of free boundaries in degenerate quenching problems (with D.J. Araújo and J.M. Urbano), submitted.
 A free boundary problem with fractional diffusion (with D. Marcon), submitted.
A free boundary problem with fractional diffusion (with D. Marcon), submitted.
 Higher Hölder regularity for degenerate elliptic PDEs with data in Morrey spaces (with G. Di Fazio and J.M. Urbano), to appear in Ann. Mat. Pura Appl.
Higher Hölder regularity for degenerate elliptic PDEs with data in Morrey spaces (with G. Di Fazio and J.M. Urbano), to appear in Ann. Mat. Pura Appl.
 The fractional Laplacian: a primer, Coimbra Mathematical Texts, Springer Cham, to appear.
The fractional Laplacian: a primer, Coimbra Mathematical Texts, Springer Cham, to appear.
 Interacting free boundaries in obstacle problems (with D.J. Araújo), Interfaces Free Bound. (2025).
Interacting free boundaries in obstacle problems (with D.J. Araújo), Interfaces Free Bound. (2025).
 Fully nonlinear dead-core systems (with D.J. Araújo), J. Funct. Anal. 287 (2024), no. 9, Paper No. 110586, 23 pp.
Fully nonlinear dead-core systems (with D.J. Araújo), J. Funct. Anal. 287 (2024), no. 9, Paper No. 110586, 23 pp.
 Sharp regularity for singular obstacle problems (with D.J. Araújo and V. Voskanyan), Math. Ann. 387 (2023), 1367-1401.
Sharp regularity for singular obstacle problems (with D.J. Araújo and V. Voskanyan), Math. Ann. 387 (2023), 1367-1401.
 An optimal Liouville theorem for the porous medium equation (with D.J. Araújo), Arch. Math. (Basel) 118 (2022), 427-433.
An optimal Liouville theorem for the porous medium equation (with D.J. Araújo), Arch. Math. (Basel) 118 (2022), 427-433.
 Reaction-diffusion equations for the infinity Laplacian (with N. Diehl), Nonlinear Anal. 199 (2020), 111956, 12pp.
Reaction-diffusion equations for the infinity Laplacian (with N. Diehl), Nonlinear Anal. 199 (2020), 111956, 12pp.
 Fully nonlinear integro-differential equations with deforming kernels (with L. Caffarelli and J.M. Urbano), Comm. Partial Differential Equations 45 (2020), 847-871.
Fully nonlinear integro-differential equations with deforming kernels (with L. Caffarelli and J.M. Urbano), Comm. Partial Differential Equations 45 (2020), 847-871.
 Singularly perturbed fully nonlinear parabolic equations and their asymptotic free boundaries (with G.C. Ricarte and J.M. Urbano), Rev. Mat. Iberoam. 35 (2019), 1535-1558.
Singularly perturbed fully nonlinear parabolic equations and their asymptotic free boundaries (with G.C. Ricarte and J.M. Urbano), Rev. Mat. Iberoam. 35 (2019), 1535-1558.
 Sharp regularity estimates for quasilinear evolution equations (with M.D. Amaral, J.V. da Silva and G.C. Ricarte), Israel J. Math. 231 (2019), 25-45.
Sharp regularity estimates for quasilinear evolution equations (with M.D. Amaral, J.V. da Silva and G.C. Ricarte), Israel J. Math. 231 (2019), 25-45.
 Homogenization of obstacle problems in Orlicz-Sobolev spaces (with D. Marcon and J.F. Rodrigues), Port. Math. 75 (2018), 267-283.
Homogenization of obstacle problems in Orlicz-Sobolev spaces (with D. Marcon and J.F. Rodrigues), Port. Math. 75 (2018), 267-283.
 A free boundary optimization problem for the
A free boundary optimization problem for the 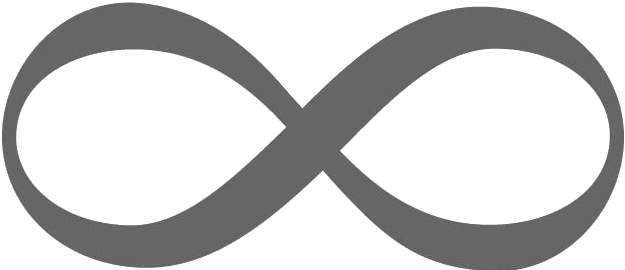 -Laplacian (with J.M. Urbano), J. Differential Equations 263 (2017), 1140-1159.
-Laplacian (with J.M. Urbano), J. Differential Equations 263 (2017), 1140-1159.
 Cavity type problems ruled by infinity Laplacian operator (with G.C. Ricarte and J.V. Silva), J. Differential Equations 262 (2017), 2135-2157.
Cavity type problems ruled by infinity Laplacian operator (with G.C. Ricarte and J.V. Silva), J. Differential Equations 262 (2017), 2135-2157.
 Optimal design problems with fractional diffusions (with E.V. Teixeira), J. Lond. Math. Soc. (2) 92 (2015), 338-352.
Optimal design problems with fractional diffusions (with E.V. Teixeira), J. Lond. Math. Soc. (2) 92 (2015), 338-352.
 On the regularity of the free boundary for quasilinear obstacle problems (with S. Challal, A. Lyaghfouri and J.F. Rodrigues), Interfaces Free Bound. 16 (2014), 359-394.
On the regularity of the free boundary for quasilinear obstacle problems (with S. Challal, A. Lyaghfouri and J.F. Rodrigues), Interfaces Free Bound. 16 (2014), 359-394.
 On the two obstacles problem in Orlicz-Sobolev spaces and applications (with J.F. Rodrigues), Complex Var. Elliptic Equ. 56 (2011), 769-787.
On the two obstacles problem in Orlicz-Sobolev spaces and applications (with J.F. Rodrigues), Complex Var. Elliptic Equ. 56 (2011), 769-787.
 A one-phase parabolic problem with a free boundary in a convex ring (with M. Poghosyan), J. Contemp. Math. Anal. 44 (2009), 192-204.
A one-phase parabolic problem with a free boundary in a convex ring (with M. Poghosyan), J. Contemp. Math. Anal. 44 (2009), 192-204.
 PhD thesis: Obstacle type problems in Orlicz-Sobolev spaces, University of Lisbon, UT Austin | Portugal CoLab program, 2013.
PhD thesis: Obstacle type problems in Orlicz-Sobolev spaces, University of Lisbon, UT Austin | Portugal CoLab program, 2013.
 The fractional Laplacian uncovered (with D. dos Prazeres and A. Sobral), Brazilian Mathematics Colloquium, vol. 35-10, IMPA, 2025.
The fractional Laplacian uncovered (with D. dos Prazeres and A. Sobral), Brazilian Mathematics Colloquium, vol. 35-10, IMPA, 2025.
 Improved regularity for a nonlocal dead-core problem (with D. dos Prazeres and J.M. Urbano), submitted.
Improved regularity for a nonlocal dead-core problem (with D. dos Prazeres and J.M. Urbano), submitted.
 Geometric properties of free boundaries in degenerate quenching problems (with D.J. Araújo and J.M. Urbano), submitted.
Geometric properties of free boundaries in degenerate quenching problems (with D.J. Araújo and J.M. Urbano), submitted.
 A free boundary problem with fractional diffusion (with D. Marcon), submitted.
A free boundary problem with fractional diffusion (with D. Marcon), submitted.
 Higher Hölder regularity for degenerate elliptic PDEs with data in Morrey spaces (with G. Di Fazio and J.M. Urbano), to appear in Ann. Mat. Pura Appl.
Higher Hölder regularity for degenerate elliptic PDEs with data in Morrey spaces (with G. Di Fazio and J.M. Urbano), to appear in Ann. Mat. Pura Appl.
 The fractional Laplacian: a primer, Coimbra Mathematical Texts, Springer Cham, to appear.
The fractional Laplacian: a primer, Coimbra Mathematical Texts, Springer Cham, to appear.
 Interacting free boundaries in obstacle problems (with D.J. Araújo), Interfaces Free Bound. (2025).
Interacting free boundaries in obstacle problems (with D.J. Araújo), Interfaces Free Bound. (2025).
 Fully nonlinear dead-core systems (with D.J. Araújo), J. Funct. Anal. 287 (2024), no. 9, Paper No. 110586, 23 pp.
Fully nonlinear dead-core systems (with D.J. Araújo), J. Funct. Anal. 287 (2024), no. 9, Paper No. 110586, 23 pp.
 Sharp regularity for singular obstacle problems (with D.J. Araújo and V. Voskanyan), Math. Ann. 387 (2023), 1367-1401.
Sharp regularity for singular obstacle problems (with D.J. Araújo and V. Voskanyan), Math. Ann. 387 (2023), 1367-1401.
 An optimal Liouville theorem for the porous medium equation (with D.J. Araújo), Arch. Math. (Basel) 118 (2022), 427-433.
An optimal Liouville theorem for the porous medium equation (with D.J. Araújo), Arch. Math. (Basel) 118 (2022), 427-433.
 Reaction-diffusion equations for the infinity Laplacian (with N. Diehl), Nonlinear Anal. 199 (2020), 111956, 12pp.
Reaction-diffusion equations for the infinity Laplacian (with N. Diehl), Nonlinear Anal. 199 (2020), 111956, 12pp.
 Fully nonlinear integro-differential equations with deforming kernels (with L. Caffarelli and J.M. Urbano), Comm. Partial Differential Equations 45 (2020), 847-871.
Fully nonlinear integro-differential equations with deforming kernels (with L. Caffarelli and J.M. Urbano), Comm. Partial Differential Equations 45 (2020), 847-871.
 Singularly perturbed fully nonlinear parabolic equations and their asymptotic free boundaries (with G.C. Ricarte and J.M. Urbano), Rev. Mat. Iberoam. 35 (2019), 1535-1558.
Singularly perturbed fully nonlinear parabolic equations and their asymptotic free boundaries (with G.C. Ricarte and J.M. Urbano), Rev. Mat. Iberoam. 35 (2019), 1535-1558.
 Sharp regularity estimates for quasilinear evolution equations (with M.D. Amaral, J.V. da Silva and G.C. Ricarte), Israel J. Math. 231 (2019), 25-45.
Sharp regularity estimates for quasilinear evolution equations (with M.D. Amaral, J.V. da Silva and G.C. Ricarte), Israel J. Math. 231 (2019), 25-45.
 Homogenization of obstacle problems in Orlicz-Sobolev spaces (with D. Marcon and J.F. Rodrigues), Port. Math. 75 (2018), 267-283.
Homogenization of obstacle problems in Orlicz-Sobolev spaces (with D. Marcon and J.F. Rodrigues), Port. Math. 75 (2018), 267-283.
 A free boundary optimization problem for the
A free boundary optimization problem for the  -Laplacian (with J.M. Urbano), J. Differential Equations 263 (2017), 1140-1159.
-Laplacian (with J.M. Urbano), J. Differential Equations 263 (2017), 1140-1159.
 Cavity type problems ruled by infinity Laplacian operator (with G.C. Ricarte and J.V. Silva), J. Differential Equations 262 (2017), 2135-2157.
Cavity type problems ruled by infinity Laplacian operator (with G.C. Ricarte and J.V. Silva), J. Differential Equations 262 (2017), 2135-2157.
 Optimal design problems with fractional diffusions (with E.V. Teixeira), J. Lond. Math. Soc. (2) 92 (2015), 338-352.
Optimal design problems with fractional diffusions (with E.V. Teixeira), J. Lond. Math. Soc. (2) 92 (2015), 338-352.
 On the regularity of the free boundary for quasilinear obstacle problems (with S. Challal, A. Lyaghfouri and J.F. Rodrigues), Interfaces Free Bound. 16 (2014), 359-394.
On the regularity of the free boundary for quasilinear obstacle problems (with S. Challal, A. Lyaghfouri and J.F. Rodrigues), Interfaces Free Bound. 16 (2014), 359-394.
 On the two obstacles problem in Orlicz-Sobolev spaces and applications (with J.F. Rodrigues), Complex Var. Elliptic Equ. 56 (2011), 769-787.
On the two obstacles problem in Orlicz-Sobolev spaces and applications (with J.F. Rodrigues), Complex Var. Elliptic Equ. 56 (2011), 769-787.
 A one-phase parabolic problem with a free boundary in a convex ring (with M. Poghosyan), J. Contemp. Math. Anal. 44 (2009), 192-204.
A one-phase parabolic problem with a free boundary in a convex ring (with M. Poghosyan), J. Contemp. Math. Anal. 44 (2009), 192-204.





